Newton's 3 Laws of Motion

      Over the past few weeks, we've been learning about a couple very important physics topics. We've covered the relationships between mass, force, and acceleration; and we also went over Newton's 3 laws of motion. 

Fan Cart Lab:

      In this lab, we covered the relationships between force, mass, and acceleration by using a fan cart to measure accelerations. We began by finding the force of our fan cart set on high by placing a force probe on our track. (Shown below)

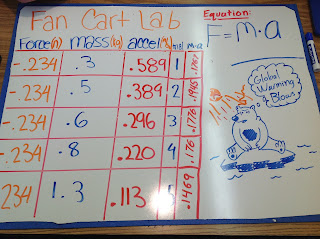
      We found our force value to be -0.234 which we would later use to create our equation. We then proceded by measuring the acceleration of the cart with a variety of different weights placed on it. Using the logger pro application on the computers, we found the acceleration of the cart by looking at the slope of our graph. (Shown below)

(After collecting all of our data, we were able to organize everything into a data table as shown on our whiteboard.)

      After completing our lab, we used our data values for force, mass, and acceleration to come up with the equation F = ma. (Force equals mass x acceleration) Our values for mass and acceleration didn't exactly match up with our force value when we plugged them into our equation, but after going back again to find a more exact force value, we found a better number that made more sense with our equation. We just didn't take our time when trying to find the force value the first time. 

Newton's 3 Laws of Motion:

      Aside from finding our F=ma equation, we also learned about Newton's 3 laws and how they applied to our everyday lives. 

Hover Disk Lab:

       In this lab, we spent most of the time sliding a hover disk across the floor and trying to figure out all the forces that were being felt by it. It was actually a tricky lab because we not only had to find the forces that applied to the disk, but also the forces that applied to the earth and each other. 

      For example, if I were to shove the disk over to my partner, there are lots of forces interacting that we usually don't think about. We were able to show these interactions with different diagrams.

(As we can see from the interaction diagram above, there are normal forces and gravitational forces acting between all four of the "objects" involved.)

      We continued this lab with many more different scenarios involving the hover disk. Overall this lab just helped us to better understand the relationships going on between different things in our lives.

Newton's 1st Law:

Newton's 1st law states that any object at rest or constant speed will remain at rest or constant speed. This law applied to our hover disk lab, because we saw that if we left our disk alone and didn't push it, it would stay in the same spot. This law also applied when we pushed the disk across the floor and it continued moving at a constant speed because it was feeling no friction with the floor. 

Newton's 2nd Law:

Newton's 2nd law states that acceleration is produced when a force acts on a mass. This law applied well to our fan cart lab where we found our own force values, and then used the acceleration from the fan cart, to arrive at the equation, F = ma.

Newton's 3rd Law:

Newton's 3rd law states that for every action, there is an equal and opposite reaction. Now we didn't have any labs that specifically applied to this law, but we talked about it a lot during class. For example, if someones hand were to press down on a table, that same force being applied to the table is also being equally applied to that hand. 

Real World Application:

      There are actually a lot of real world applications that we could take from these past few weeks. However, I think that Newton's 3rd law is the most common law that all of us have experienced without realizing it. 

      One of the best examples I can think of comes when watching baseball games. We don't think about it, but when the player's bat collides with the baseball, that is an example of equal and opposite reactions. Or when a football player kicks a field goal, there are equal and opposite reactions between his foot and the ball. But above all, the best visual example of Newton's 3rd law comes from the Newtons Cradle.

 

Impulse Lab

Lab Work:
      In our most recent lab, we performed experiments using the equation for momentum,
P=mV, and the equation for Impulse, J=P(after) - P(before). 

      Our actual test consisted of crashing a cart with a metal ring into a force probe stand with a metal ring. The force probe helped us to calculate the force of the collision. And at the end of the track we also placed a sonar device, which allowed us to calculate the velocity of our cart before and after the collision. We had to perform this test a few times in order to get the data we needed to use. 

(The graph above shows the data we collected from our collision. The blue bar on the bottom was the data we used to find our velocity before and after the collision.)

      Our whiteboard above shows the calculations we made after completing our lab. We used the momentum equation, P=mV to find the momentum of the cart before and after the collision. The mass remained the same at 0.25k. Before the collision, the velocity was 0.3714m/s, and after the collision the velocity was -0.3421m/s. After we had our values for momentum, we subtracted the momentum before the collision from the momentum after the collision. This value would be our impulse for the collision. 

(We also calculated our percent error as shown on the board and found that we had a twenty percent difference.)

Real World Application:

      In our world today, one example of this lab would be a car crash. Now this type of car crash wouldn't involve another car like some of our previous labs. Instead of a car crashing into another vehicle, this car would crash into a wall or something immovable. 

Crash test cars are an excellent example of this concept in real life. By crashing a car into a solid wall, people are able to record impulse, momentum, velocity and many other important factors just like we did in our impulse lab. 

Collisions Lab

Big Question:

"What is a better conserved quantity - momentum, or energy?"
-After completing our collisions lab and collecting our data, we found that momentum is a better conserved quantity as opposed to energy. With energy, both cars start out with kinetic energy, but when they collide, the energy is transfered to heat, or possibly friction. 

Lab Work:
      We began our lab by setting up two cars on a track facing each other. On either end of the track itself we had sonar sensors which would allow us to calculate the velocity of the cars. Our first test was "elastic" meaning that each car would have springs colliding with each other on the ends of the cars. The red car was stationary and we rolled the blue car into it. The blue car stopped moving and the red car was pushed down the track.This was our first test for the "elastic" collision.

      Our second test was an "inelastic" collision where we took the springs away from the cars. This time, when we ran the blue car into the red car, both cars stayed together and continued rolling down the track. 

      Our data involving momentum, energy, and velocity are recorded below:

(We used the formula, P = mv, to calculate momentum, and the formula, K = 0.5mV^2, to calculate the energy.)

Percent Difference:

      This whiteboard shows how we calculated the percent difference of energy and momentum. We calculated the percent difference of the energy and momentum by first subtracting the "before" number from the "after" number. Then we divided that number by the average of the two numbers. We then took that number and multiplied it by 100 to give us our percent difference value.

Real World:

      In our world today, one of the most obvious examples is a car crash. When one car crashes into the other, both of the cars continue moving a little. The kinetic energy of the cars is transfered into heat or friction and the momentum of the cars is conserved. This is an example of an "inelastic" collision as performed in our lab. 

Rubber Band Cart Launcher Lab

Big Question:
"How are energy and velocity related?"
-From our lab, we were challenged to work with many different equations that helped us relate energy to velocity. We used the equation for spring potential energy, kinetic energy, and gravitational potential energy. By using these various equations, we were able to calculate values and relationships between energy and velocity.


Lab:
This lab was like a sequel to our previous lab with the rubber bands. We used the same setup with the air track and rubber band. Only this time instead of finding the force required for the rubber band, we were testing an entirely new concept of velocity. 
      We used equations we learned in class like the kinetic energy equation, K = 1/2mV^2, to help us calculate the velocity. 
      Our testing was basically just pulling the red glider back a certain distance in meters, then letting it go on the air track. After being released, the glider would pass under a velocity recorder. We connected this recorder to our labquest devices and were able to find the speed of the glider being pulled back at various distances. 

(Whiteboard showing our data plotted on a graph using Vernier Graphical Analysis.)

      After gathering all our data, we averaged it, squared it, and from there we could plug it into our equation to find the energy if we wanted to. This lab wasn't really about finding the energy, it was mainly just about getting practice with velocity and how it can relate to different forms of energy.

Today's World:

      In our world today, these concepts of potential energy, kinetic movement, and velocity can be easily seen at any amusement park. Roller coasters may seem like fun and games, but there is actually a great deal of physics involved in these rides. When the car is stalled at the top of a hill on the roller coaster, it still has potential energy. When it slowly creeps forward and starts to plummet down the track, the potential energy is converted to kinetic energy and this movement carries the car around the rest of the track because no energy is lost, it only changes forms.

Rubber Band Lab

Big Question:
"How does the force it takes to stretch a rubber band depend on the AMOUNT by which you stretch it?"
-After completing this lab, we found that by stretching a rubber band over a distance, the more force is required the farther you stretch.

      We began this lab by putting a rubber band on an air track:

(We didn't use the red piece^)

      Our testing involved stretching the rubber band over various distances and recording how much force was required to hold the rubber band AFTER it was stretched. We were creating potential energy, so we wanted to measure how much force was required to hold the rubber band, not pull it. 

      We used a force probe to record the force and completed two different types of tests. First we measured the force of 5 separate distances with the rubber band only single stretched; we then double stretched the rubber band and measured the same 5 distances. After all of our testing was complete, we recorded our data and found that the amount of required force to hold the rubber band was increasing.

   Single band:                   Double Band:           

1cm - 0.90N                    1cm - 5.20N

 2cm - 1.05N                    2cm - 6.90N

 3cm - 2.10N                    3cm - 7.78N

   4cm - 2.75N                    4cm - 10.08N

   5cm - 3.60N                    5cm - 11.32N

In our world today, we can see this principle being used especially by hunters. Many hunters prefer to use whats known as a compound bow. These are very heavy bows and require great amounts of force to pull back. The farther you pull back the string, the more force you need. When you pull the string back all the way, you've created potential energy.

Fun Fact: Compound bows use pulley systems. This means that you can pull the string back the same distance as a normal bow, but use less force because of the pulley system.

Pyramid Lab

      In this lab, we were using simple car and ramp systems to measure the relationships between force, distance, and work.

      Our experiment started out with a 750g car. We placed it on a ramp and attached a force probe to it. For our first test, we pulled the car up the ramp for a distance of 1.4m. (This required 0.36N of force) For the second test, we made the ramp steeper, and dragged the car a distance of 1m. (This required a force of 0.53N) For our final test, we made the ramp even steeper, and pulled our car a distance of 0.8 meters. (Requiring a force of 0.65N)

      After we gathered all our data, we found that the work required to pull the car in all three tests was roughly the same. From our three tests we averaged 0.52J of work was required. So basically, we put in the same amount of effort each time, the only thing that changed was how far the car traveled up the ramp.

      In our world today we can apply this notion to anything that involves a ramp. Movers for example, use ramps all the time to load and unload heavy objects. From our tests we noticed that the longer the distance of the ramp, the less force was required to pull the car. Movers use this notion when unloading couches or refrigerators. Its much easier to push a couch up a ramp instead of lifting it straight off the ground 5 feet in the air.

(The longer the ramp, the less force you need to pull the object.)

(These movers probably couldn't lift that piano straight into the truck. Thats why they're using a ramp.)

Simple Machines: Pulley Lab

      In our most recent lab, we spent time working with various pulley systems to help understand the relationship between force and distance.

      During the first few days of the lab, we constructed a simple pulley machine using only one pulley, a brass weight or 200g, and a length of string. We basically just did a few tests to see how much force was required to move the weight 10cm with and without a pulley.

  Our results ending up showing us that it required roughly 1N to move the weight 10cm with a pulley, and roughly 2N to move the weight 10cm without a pulley. We can conclude from these short tests that with one simply pulley, we only have to use half of our force to move an object that would normally take twice the force. This concept is very practical in the real world. Today workers everywhere use pulleys to get their job done with less effort.

      After completing the work with out simple pulley systems, we moved on to more complex pulley systems with more strings. A very useful tool we used in this lab was the force probe. This device allowed us to measure the amount of force being used to pull the brass weight with various pulley systems. 

 

(We connected these force probes to our labquest devices and were able to read how much force was being used to lift our 200g mass.)

      We used a simple pulley, one with two strings, and one with four strings. As we completed our tests we found out that less force was required with more stings/pulleys. For example, with a simple pulley, the required force was almost 2N. But with a four string pulley, the required force was 0.5N. From our work it was clear to us that the more complex pulley system we had, the less amount of force was required.

(This whiteboard shows our work in graph/data table form. After graphing all of our work we were able to see that each test took up the same area on the graph.)

After completing this lab, I was able to see how these simple pulley systems play vital roles in the real world. Workers today, especially at docks and construction sites, use pulley systems to lift heavy weights and get their jobs done easier. 

 

(This is an example of a simple pulley system used on boats to lift heavy cargo onto their decks)

(This crane also has lots of pulley systems which allow it to lift items normally to heavy for the amount of force it uses)

Mass vs. Force Lab

      In the past few days of Physics class, we've been working on a lab that deals with mass and force. In the actual lab itself, I, along with 3 other partners, would put various "brass masses" on a scale and record how how many Neutons each weight would have according to its mass. 

      In our lab we found that the number of Newtons was directly proportional to the mass of a weight. For example, if one weight had a mass of 0.5kg, then it would be equal to 5N; or if a weight had a mass of 1kg, then it would be equal to 10N. We plugged our data into the slope formula and the equation of a line formula and ultimately we came up with the equation f = 10m. (force = 10 X mass)With this equation we would be able to predict how many Newtons a weight would have without going to all the trouble of putting it on the scale.
      In the real world today, gravity is used in almost everything we do; it's what holds us down to the planet. In our lab, gravity was a key factor that helped us determine the force of a certain object. When holding our scale still, gravity would constantly be pulling down on our brass masses which would let us know how many Newtons each mass had. Just as gravity was pulling down on our weights, it also pulls down on everything else on the planet. For example, if we jumped out of a plane, gravity would pull us down. Just as if we held up a weight, gravity would also pull it down.

      This lab was overall very helpful and gave all of us good experience working with data, graphs, and scientific formulas. I can now better understand the connection between how gravity is used in experiments, and how it applies to everything around me.